mamech
Full Member level 3

- Joined
- Nov 9, 2010
- Messages
- 176
- Helped
- 0
- Reputation
- 0
- Reaction score
- 0
- Trophy points
- 1,296
- Activity points
- 3,135
Hello everyone
I want to design a second order low pass filter, but I need to select values of resistances and capacitances so I get underdamped oscillation for a step input.
I have read that this can be done using RLC circuit, but I want to design it using two cascaded RC first order filters, but i could not manage to do this. As I understand, the matter is just a selection of parameters for having damping ratio < 1 , but I could not get any values that achieve the goal.
I wonder, is it possible to design a second order filter using two RC circuits while making the system underdamped??
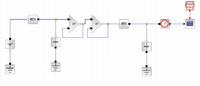
I want to design a second order low pass filter, but I need to select values of resistances and capacitances so I get underdamped oscillation for a step input.
I have read that this can be done using RLC circuit, but I want to design it using two cascaded RC first order filters, but i could not manage to do this. As I understand, the matter is just a selection of parameters for having damping ratio < 1 , but I could not get any values that achieve the goal.
I wonder, is it possible to design a second order filter using two RC circuits while making the system underdamped??


