mr_monster
Member level 4

- Joined
- May 10, 2012
- Messages
- 79
- Helped
- 0
- Reputation
- 0
- Reaction score
- 0
- Trophy points
- 1,286
- Activity points
- 1,964
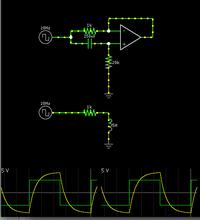
I would like to make a simulated inductor which is tune-able using an opamp implementation of an opamp. I could find a good source for the design process so anything like that would be greatly appreciated. Thanks!