Full wave rectifier using a capacitor input filter

[bila ljaved]

guys this is my another question, I hope all you will help me inspite of not getting screwed up because there are many more questions to come.

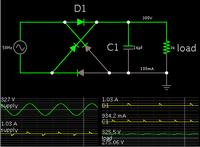
A Full wave rectifier using a capacitor input filter is to supply 100mA at 300V, What will the secondary voltage of the transformer?

If the capacitor has a value of 16µF, the % ripple will be??

 

[zorro]

Hi,

For that kind of designs and analysis, Schade curves are very useful.
I posted them in this forum some time ago. Please follow this link:

https://www.edaboard.com/threads/223643/#post955542

Regards

Z

 

[kam1787]

Vac = 300 / 1.44 + 1.4

 

[bila ljaved]

"

Hi,

For that kind of designs and analysis, Schade curves are very useful.
I posted them in this forum some time ago. Please follow this link:

https://www.edaboard.com/threads/223643/#post955542

Regards

Z"

DEAR ZORRO!

HOW CAN I FIND OUT THE TRANSFORMER SECONDARY VOLTAGE FROM THESE SHADE CURVES FOR THE ABOVE GIVEN DATA, CAN YOU PLEASE EXPLAIN....?

Vac = 300 / 1.44 + 1.4

DEAR KAM 1787,

YOUR EXPLANATION YIELDS AN ANSWER OF 105.68V, BUT ACTUALLY THE ANSWER IS 234-0-234 V.

BUT I CAN NOT FIND OUT THE EXACT ANSWER, WHAT IS THE METHOD TO FIND OUT THE ANSWER OF SUCH QUESTION......?

 

[kam1787]

"

Hi,

HOW CAN I FIND OUT THE TRANSFORMER SECONDARY VOLTAGE FROM THESE SHADE CURVES FOR THE ABOVE GIVEN DATA, CAN YOU PLEASE EXPLAIN....?

DEAR KAM 1787,

YOUR EXPLANATION YIELDS AN ANSWER OF 105.68V, BUT ACTUALLY THE ANSWER IS 234-0-234 V.

BUT I CAN NOT FIND OUT THE EXACT ANSWER, WHAT IS THE METHOD TO FIND OUT THE ANSWER OF SUCH QUESTION......?

I do not know how you do math, but I get 209.7VAC.

Anyways, I had assumed you were asking about full-wave bridge rectification. because you failed to state a centre tapped secondary winding is used. If you fail to provide the correct information, you can not expect a correct answer.



This link should have want you are puzzled about https://www.electronics-tutorials.ws/diode/diode_6.html

It was easy to find.

 

[bila ljaved]

dear kam,
no actually am not sure about this too what 234-0-234 V meant. i was searching the meaning all over the web.
any how dear still if the answer is 209.7 VAC it is not the required one.

 

[kam1787]

required by who?

234-0-234 V refers to the windings - refer to the link previously given

 

[BradtheRad]

A simulation is helpful to check the math...



The load is 3k ohms, to draw 100mA.

Values were adjusted so that load voltage was centered around 300 VDC.

The rectified sine peaks need to be 327 V.
Divide by 1.414, yields 231 VAC nominal.

The capacitor is 16 uF. Ripple is about 17%.

 

[FvM]

Yes, about 230 VAC seems to be right. And 234-0-234 V suggests a center tap instead of bridge full wave rectifier.

 

[bila ljaved]

A simulation is helpful to check the math...



The load is 3k ohms, to draw 100mA.

Values were adjusted so that load voltage was centered around 300 VDC.

The rectified sine peaks need to be 327 V.
Divide by 1.414, yields 231 VAC nominal.

The capacitor is 16 uF. Ripple is about 17%.

Dear Bratherad,

please can you mention the math that you carried out to find the peak value of rectified sine wave that is 327 VAC?

also please show how you found that the ripple % is 17%?
PLEASE SHOW IN MATHEMATICS.
Thanks.

- - - Updated - - -

required by who?

234-0-234 V refers to the windings - refer to the link previously given

required means that the required answer of the above posted question by me is 234-0-234 V

 

[BradtheRad]

Dear Bratherad,

please can you mention the math that you carried out to find the peak value of rectified sine wave that is 327 VAC?

also please show how you found that the ripple % is 17%?
PLEASE SHOW IN MATHEMATICS.
Thanks.

This is a middle situation between no load and a heavy load.

With no load you would want 211 VAC supply, to obtain 300 VDC out.
With a heavy load you would want 300 VAC supply.

To tell the truth, I have not worked out the math for in-between loads. I believe it involves finding the amount of area under the sine wave, which is equal to the amount of power taken during the dead time. It involves calculus, and for us to work it out would be tedious. I cheated and used the simulator. I experimented with values until I got 300V at the load.

The power supply does not need to do math, of course. It automatically finds the break-even point.

---------------------------

To figure out the ripple, I looked at the waveform on the load. It goes 25V above and below 300V. A total of 50V. This equals 1/6 of 300, or 17%.

There is a formula to calculate ripple. One method uses the RC time constant:

16uF times 3000 ohms is .048 (the time constant).

It means the capacitor will lose 63% of its charge in .048 sec.

Dead time is 1/100 of a second. In that time the capacitor will lose 21% of its charge (.048 x .01). (We are making it easy on ourselves by pretending the discharge curve is a straight line even though it is not.)

So ripple is 21%. Again, an approximate figure.

 

[zorro]

DEAR ZORRO!

HOW CAN I FIND OUT THE TRANSFORMER SECONDARY VOLTAGE FROM THESE SHADE CURVES FOR THE ABOVE GIVEN DATA, CAN YOU PLEASE EXPLAIN....?

Hi, bila ljaved

Let me show how to use the curves in the case you presented.
Look at the figure on the second page (lebelled as Fig.14).

You must supply Idc=100mA at Edc=300V, so the load is Rload=Edc/Idc=3000 ohms.

Let's assume that the total series resistance (the equivalent resistance of the transformer plus the dynamic resistance of the two diodes in the case of the full-wave bridge) is Rs=30 ohms, so Rs/Rload=1%.

Now, if the frequency is 50 Hz and C=16 uF, ω*Rload*C=15.1 .
With this abscissa, for the curve corresponding to the found Rs/Rload=1% we get Edc/Et(max)=91% (ordinate at the left).

As Edc=300V, we obtain Et(max)=300V/0.91, but for silicon diodes we shuold add about 1.5V giving Et(max)=331V. This is the peak voltage, so Et(rms)=Et(max)/sqrt(2)=234V.

(Observe that if C is big enough we are in the right flat part of the curve ans Et would be independent of C. This happens because the ripple is much smaller than Edc.)

Now, with the figure at page 4 (labelled Fig.16) we obtain the ripple.
For the full-wave circuit and Rs/Rload=1% we must use the third curve counting from the bottom (see the table CURCUIT-PARAMETER).

For the abscissa ω*Rload*C=15.1, we read from the plot that the ripple is about 5.5%. So, the rms value of the ripple is 300V*0.055=16.5V .

I hope it is clear.
regards

Z